|
The Squared Square |
|
Because there is much other online information on this
topic, I have elected to share only a couple of examples. |
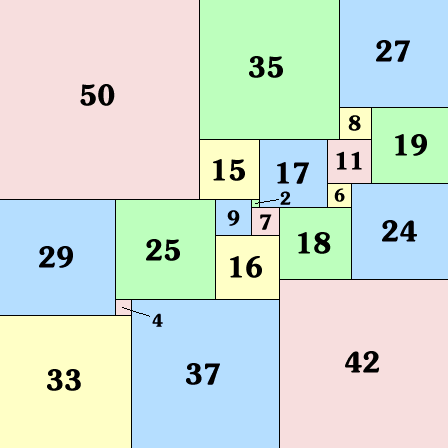
"Squaring the square" with areas of unique sizes was long
considered to be impossible. In 1939, however, R.P. Sprague
of Germany published a 55-square solution. A major
'improvement' upon that discovery was this 26-square layout,
first published by W.P. Tutte of Canada in 1940:
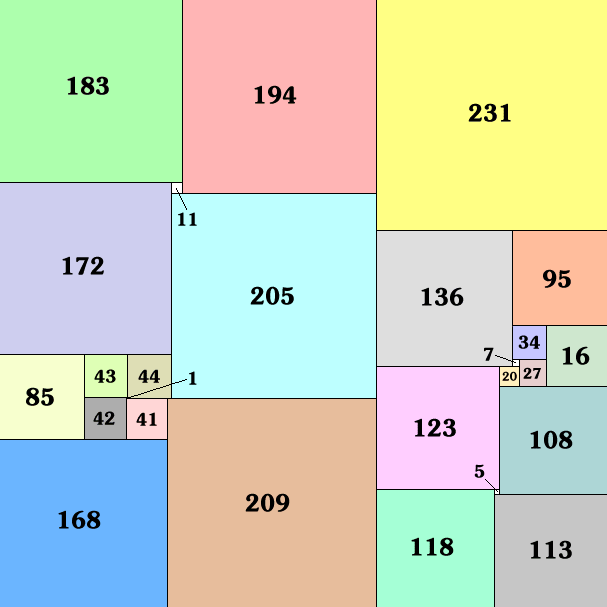
Subsequently, it has been shown that the lowest-order possible solution
is 21 squares. The matrix shown below, believed to be unique,
was discovered in 1978 by W.A. Duijvestijn of The Netherlands: